Irrational Numbers
Irrational numbers are usually expressed in the R/Q form, where the backward slash symbol represents “set minus”. Hence, it can also be written in the form of R – Q, which describes the difference between the set of real numbers and the set of rational numbers. Now keep scrolling down and let's find out what are irrational numbers in math, a list of irrational numbers, how to find them, etc.
What is an irrational number give examples?
In maths, an irrational number can be defined as:
“A real number that cannot be written in the form of fraction p/q of integers, and q is not equal to zero”
An irrational number gives the decimal expansion value that can neither be terminated nor recurring and every transcendental number is an irrational number.
Irrational numbers examples:
Examples of irrational numbers include √6, √13, √23, etc. And, if such numbers are used in arithmetic operations, it’s crucial to evaluate the values of under root first. Sometimes these values could also be recurring.
Irrational numbers meaning:
Irrational means not to have a ratio or ratio that can not be written for a particular number. It means the number that cannot be expressed other than by means of roots. Simply in other words, we can say that irrational numbers cannot be explained as the quotient of two integers.
Symbol used for an irrational number:
Generally, the symbol used to express the irrational number is “P”. The symbol P is typically used because of the connection with the real number and rational number i.e., according to the alphabetic sequence P, Q, R. But in most cases, it is expressed using the set difference of the real minus rationals, such as R- Q or R\Q.
Properties:
Since irrational numbers are the subsets of the real number, these numbers will follow the properties of the real number system. Properties of the irrational numbers are given bellow:
-
When an irrational number and rational number are added, the given result will become an irrational number. Suppose that x is an irrational number, y is a rational number, and then the addition of both numbers x+y gives an irrational number z.
Examples:
When we add two irrational numbers such as 3√5+ 4√3, a sum is an irrational number.
But, let us consider another example, (3+4√3) + (-4√3 ), the given results is 3, which is a rational number.
-
Multiplication of an irrational number with a non-zero rational number results in an irrational number.
Let us suppose that if xy=z is rational, then x =z/y is rational, disputing the assumption that x is irrational. Thus, the product xy must be irrational.
Example:
√2 * √2 = 2.
Here, √2 is an irrational number. But if it is multiplied twice a time, then the final product will be obtained as a rational number such as 2.
Therefore, we should be careful while adding and multiplying two irrational numbers since it gives results in an irrational number or a rational number.
-
There is no possibility of LCM (least common multiple) of any two irrational numbers that may or may not exist.
-
The addition or the multiplication of the same two irrational numbers can be rational
-
Irrational numbers are not closed under the multiplication process, unlike rational numbers.
However, using fraction calculator you can resolve problems regarding to rational or irrational numbers within a few seconds.
List of Irrational Numbers
Generally, the irrational number consists of Pi, Euler’s number, and the Golden ratio. Numerous square roots, as well as cube roots, are also irrational, but not all of them.
Example:
√3 is an irrational number but on the other hand, √4 is a rational number since 4 is a perfect square, such as 4 = 2 x 2 and √4 = 2, which is a rational number. You should note that infinite irrational numbers occur between two real numbers.
Example:
Consider 1 and 2, there are multiple infinitely irrational numbers between 1 and 2.
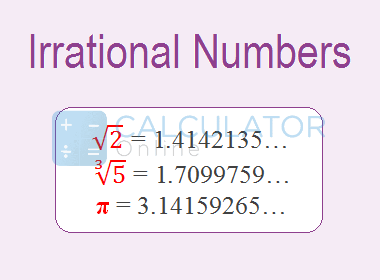
Now, let’s have a look at the values of famous irrational numbers as arranged in the table below:
|
Pi, π
|
3.14159265358979…
|
|
Euler’s Number, e
|
2.71828182845904…
|
|
Golden ratio, φ
|
1.61803398874989….
|
Irrational Number Proof
The following theorem is used to prove the irrational number
Theorem:
Let us p is a prime number and a^2 is divisible by p, (where a is any positive integer), then we can say that p also divides a.
Proof:
By using the Fundamental Theorem of Arithmetic form, the positive integer can be expressed in the form of the product of its prime number as:
a = p1 × p2 × p3……….. × pn …..(1) Where, p1, p2, p3, ……, pn define all the prime factors of a.
Squaring both the sides of equation (1),
a^2 = ( p1 × p2 × p3……….. × pn) ( p1 × p2 × p3……….. × pn)
⇒a^2 = (p1)^2 × (p2)^2 × (p3 )^2………..× (pn)2
According to the Fundamental Theorem of Arithmetic, the prime factorization of a natural number is individual, regardless for the order of its factors.
The only prime factors of a^2 are p1, p2, p3……….., pn. If p is a prime number and a factor of a^2, then p is one of p1, p2 , p3……….., pn. So, p will also be a factor of a.
Hence its proved, if a^2 is divisible by p, then p also divides a.
Prove that √2 is an irrational number
Solution:
Suppose, if possible, √2 is rational therefore it can be written in the form of p/q
Where
p,q U Z and q ≠ 0.
Suppose further that p/q is in its lowest form
Then, √2 = p/q, (q ≠ 0)
Squaring on both sides
2 = p^2/q^2
Or 2q^2 = p^2 (1)
The R.H.S. of this equation has a factor of 2. Hence its L.H.S. must have the same factor.
A prime number can be a factor of a square only if it happens at least twice in the
square. Therefore, p^2 should be of the form 4p^2
so that equation (1) takes the form:
4p^2 = 2q^2 ...(2)
i.e., 2p^2 = q^2 ....(3)
In this equation, 2 is a factor of the L.H.S. Therefore, q^2 should be of form 4q^2
So that equation 3 takes the form
2p^2 = 4q^2 i.e., p^2 = 2q^2 ....(4)
From equations (1) and (2),
p = 2p‘
and from equations (3) and (4)
q = 2q‘
∴ p/q =2p’/2q’
This result the hypothesis that p/q in its lowest form
Hence, √2 is an irrational number.
How to find an irrational number?
Now let us discuss how to find irrational numbers, we can find them with the following example of irrational numbers.
Example #1:
Which number is an irrational number or a rational number?
3, -.45678…, 6.5, √ 3, √ 2
Solution:
Rational Numbers include 3, 6.5 as these have terminating decimals.
Irrational Numbers include -.45678…, √ 3, √ 2 as these have a non-terminating non-repeating decimal expansion.
Example #2:
Check what's an irrational number or a rational number.
4, 6/11, -4.12, 0.21
Solution:
The decimal expansion of a rational number either can be terminated or repeated. Therefore ,4, 6/11, -4.12, 0.21 are all rational numbers.
Is Pi an irrational number?
Pi (π) is an irrational number since it gives a non-terminating value. The approximate value of pi (π) is 22/7. Also, the value of π is equal to 3.14159 26535 89793 23846 264
Since we know that π is also an irrational number, but when π is multiplied by π, the given result is π^2, which is also an irrational number.
∴ π x π = π^2
Are Irrational Numbers Real Numbers?
In maths, all the irrational numbers are considered as real number and these numbers should not be rational numbers. It means that irrational numbers could not be written as the quotient of two integers.
What is rational expression?
Rational expression is the ratio of two polynomials that is expressed in the form of p/q and q is not equal to zero. Moreover, you can calculate the value rational algeberic polynomials by using
rational expression calculator.