Future Value of Annuity Calculator

The future value of annuity calculator assists to provide the comprehensive values of annuity in the future dates. The future value of the annuity is computed at a regular interval which can be either monthly or yearly.

We can calculate the annuity payments, interest rates, and time periods desired. For understanding the terms it is essential to understand the concept of the annuity.

What is an annuity?

An annuity is a series of payments made at regular intervals of time periods. There are different examples of annuities like savings accounts, monthly mortgage payments, and pension payments. The future value of an annuity calculator is adjustable for finding the annuity for any time period regardless of whether we are calculating on a daily, monthly, or yearly basis.

The future value of an annuity can be made on a weekly, monthly, quarterly, and yearly basis. The future value of annuity calculator is a comprehensive measurement of annuity in the future dates. The interval can be based on the mutual understanding between the lending company and the customers.

Types of the annuities:

There are two types of annuities and we are going to discuss them one by one:

• Ordinary annuity
• Annuity due

Ordinary Annuity:

“When you make the payments at the end of each time interval then we call it the ordinary annuity”.

An ordinary annuity is a series of regular payments made at the end of each period, such as monthly or quarterly. We are using the ordinary annuity formula calculator for this type of annuity. The future value of annuity formula for ordinary annuity is as follows:

FV of Ordinary Annuity = C x [(1+i)^n – 1 / i)

Where:

C= Cash Flow Per Period

i= interest rate

n=number of payments

Example:

Now consider an example where cash flow per period is $1000, the interest rate is 5% and a number of periods for the ordinary annuity is 5 years time:

Then we get the following data:

C=$1000

i= 5%

n=5 years

Put the values of the ordinary annuity in the future value of annuity calculator.

FV of Ordinary Annuity = C x [(1+i)^n – 1 / i)

= $1000 x [(1+5%)^5 – 1 / 5%)

= $1000 x [(1+0.05)^5 – 1 / 0.05)

= $1000 x 5.53

= $ 5,525.64

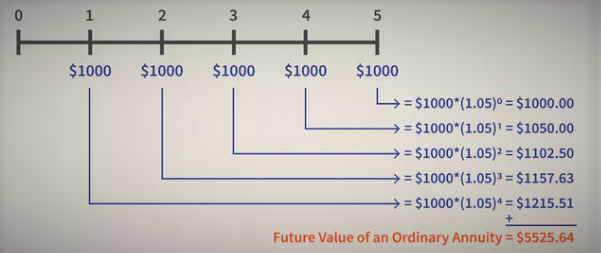
The value calculated by the ordinary annuity calculator is $ 5,525.64 after 5 years and an interest rate of 5 %.

Annuity Due:

The annuity due is paid at the beginning rather than the end of each time period.

The future value of annuity formula for annuity due is as follows, we can find the annuity due by putting the values in the future value of annuity calculator:

FV of an Annuity Due = C x [(1+i)^n – 1 / i) x (1 + i)

Example:

Let us again suppose the example mentioned for an annuity due, We have cash flow per period is $ 1000, and we want to find the annuity due of the payments. The interest rate is 5% and the number of periods for the ordinary annuity is 5 years time:

Then we get the following data:

C=$1000

i= 5%

n=5 years

Inserting the values in the Future value of annuity formula for annuity due.

FV of an Annuity Due = C x [(1+i)^n – 1 / i) x (1 + i)

= $1000 x [(1+5%)^5 – 1 / 5%)x (1 + i)

= $1000 x [(1+0.05)^5 – 1 / 0.05)x (1 + 0.05)

= $1000 x 5.53x 1.05

= $ 5801.91

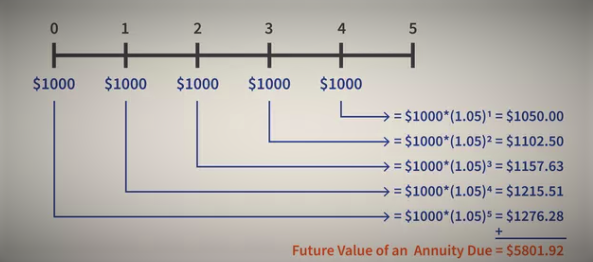
The value calculated by the annuity due calculator is $ 5801. 92 after 5 years and an interest rate of 5 %.

Terms involved in an annuity:

For understanding the future value of annuity we need to understand the preceding terms:

Period(n):

The time period between two intervals of an installment is called the future value of the annuity period. It can be yearly, monthly, or quarterly. It is denoted by the “n”.

Interest rate(i):

It is the annual rate of interest and it can be changed after a year. We can find it by dividing the interest rate by 100 as it is described as 5% or 6% etc. The future value of the annuity calculator automatically converts the values of “i” by dividing it by 100. We only have to add the values of “i” in the designated field.

Payment amount (C):

The amount paid for or the principal amount around which we are doing the compounding. It is also described as (PMT). Therefore, we are using PMT in place of “C”. The PMT stands for the Principal amount of future value of the annuity. We are using the “Cash value or “C” in the annuity payment calculator.

Growth Rate (G):

It is the growth rate of the annuity per period in the form of a percentage, which means we need to enter the growth rate as it is added to the interest rate after the time prescribed in the formulas. When you are using the future value of the annuity calculator, it is optional to prescribe the Growth Rate (G) as the interest rate remaining the same for a certain period

Payment frequency(q):

How often you are going to pay during each period of time, for yearly time periods we need to enter a value in the future value of annuity calculator =1, quarterly=4, monthly=12,daily=365, etc.

Payment types:

The payment types can be changed according to the annuity types, for the ordinary annuity, we are at the end of the installment period. The ordinary annuity formula misses the (1+i) as we are paying at the end of the installment period. For annuity due, we are paying the installment at the start of the installation time. We do add the term (1+i) as we are paying at the start of the installment period. For annuity due measurements we do require a future value annuity due calculator.

Future values (FV):

The future value or FV is the value of the present values of cash flows. We insert the values in the future value of the annuity calculator to realize the future value of the present amount. The future value of an annuity is calculated against the number of time intervals.

The Future Value of the Annuity table:

We are presenting a table by inserting the values of $1 per period (n) changes from 1 to 50 and “i” changes from 1% to 5%. We have inserted the values in the future value of the annuity calculator and rechecked all the values. The value starts from the time period “1” to “50” and the interest rate starts from 1% to 5%.

All these values are for the ordinary annuity and it is best to put the values in the future value of ordinary annuity calculator. Try to calculate for other values and time “i” and “n”.

Working on future value of annuity calculator:

The working pattern of the annuity calculator math is described as follows:

Input:

• Enter the values in the designated field.
• Enter the principal amount, the interest rate in the field
• Enter the number of periods and annuity type

Output:

When we are utilizing the future value of an annuity calculator, we are going to find the detailed output of annuity.

• The future values of annuity displayed
• Number of interest periods
• The interest rate was applied.

FAQs:

What is an ordinary annuity example in common life?

An example of the ordinary annuity is the Home mortgage, for which the homeowner is bound for the payments at the end of each month according to the decided rate of interest. The future value of ordinary annuity calculator is used to implement the ordinary annuity.

What is an annuity due in common life?

The monthly bills such as rent, car payments, and cell phone payments are done at the start of the month. The insurance amount payment is a typical example of the annuity due and we need to use the annuity due calculator to find the monthly installment.

Do annuities count as the income of a person?

The annuity is fully taxable income, when you receive an annuity you are actually purchasing an asset and it is a taxable income.

What kind of annuities are best for the seniors?

Immediate annuities for senior citizens tend to be the best annuities for seniors because they begin paying out within 12 months of purchase.

Conclusion:

Annuities are a reliable source of income over a period of time and especially for the people after retirement. It can be insurance products and equity investments. The annuity helps to balance the financial benefits for a person and also for companies. The future value of an annuity can be a source of income for the deceased person. The future value of the annuity calculator is efficient in finding the future value of the annuity.

References:

From the source of investopedia.com: Future value of annuity, Examples of annuity 

From the source of Wikipedia:Time value of money, Present value, future value

From the source of smallbusiness.chron.com:Annuity Basics, Future Value of Annuity Formula