Equation of a Line Calculator

The equation of a line calculator illustrates the vertical change divided by the horizontal change along with graphical illustrations. We also describe it as the rise over the run change in the Cartesian coordinates.

So let’s move on to know more about the line equation and its determination scenario!

Stay Focused!

What is the Equation of a Line?

The equation of a line in an algebraic form represents the set of points that together form a line in a coordinate system. Many points join together represented as a complete line and we are only finding two points to draw a line in the Cartesian coordinates.

Types of Equation of Line:

There are 3 common types of the Equation of line with a slope. We can find the equation of a line in its general forms and their corresponding relationship with these line equations as given below:

• Point Slope Form
• Slope Intercept Form
• Standard Form

Point Slope Form:

The general equation in the point-slope form can be written as:
y – y1 = m(x – x1)
Where
(x1, y1) are points on the line
“m” is the slope of the line

Point Slope Form: In the point-slope form, we only need only one point ( x1,y1) to find the equation of a line in point-slope form.
When we have only one point of the ( x1,y1) and the values of the slope “m”.
It can be convenient to put the values of one point & slope (m) in the equation of line calculator.

We can be defined in the equation of a line that passes through two points in the above-mentioned calculators. The point-slope calculator is best to unveil and draw the equation of the line.

Slope Intercept Form:

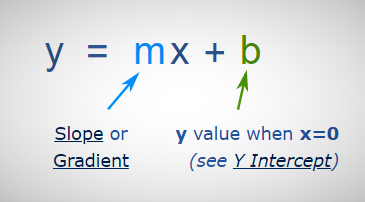
The Slope-Intercept Form can be written in the form:
y = mx + c
Where
“m” is the slope of the line
“c” is the y-intercept of the equation of the line

 Slope Intercept Calculators are just too useful in finding the Slope-Intercept Form.

Standard Form:

The Standard Form of the equation for a line can be written as:

Ax + By = C

We can also write the standard like this also:

 Ax + By + C = 0 

Where 

A, and B are the coefficient of the variables x, y

“C” is the Y-intercept of the line.

The Standard Form of the equation can be illustrated well by the Standard Form Calculator.

The Examples of All Form of Equation of line:

We unveil the whole concept of the Equation of line by depicting the practical examples of the Point slope form, Slope intercepts form and the Standards form respectively.

Example of Point Slope Form:

Let’s consider one point each along the x-axis and y-axis (3,5) and the slope  “m” is equal to 6. We can write an equation of the line that passes through points(3,5) and has a slope “6”. We know how to find the equation of a line Point Slope Form, so it can be simple how to find the equation of a line with one point and slope?

                                        y – y1 = m(x – x1)

Putting the values in the equation of the line in  Point Slope Form:                                       

                                          y – 5= 6(x – 3)

This is the equation of the line in the Point-Slope Form:

Example of Slope-Intercept Form:

Now consider the slope of the line “m” is 6 and the y-intercept “c” is 8. Then the equation of the line in the Slope-Intercept Form:

                                          y = mx + c 

Putting the values in the Slope-Intercept Form equation, we get:

                                            y=  6x+8

Example of Standard Form:

You can use the point-slope form or slope intercept form equation to convert it into the Standard Form:

You should be aware we can’t use the fractions or decimals as the coefficient of the “x”. It means the coefficient of the “x” should be just positive:

Take the Slope intercept form:   

                                                       y=  6x+8

 Now we apply the operation: 

                                                     ⇒    6x+8-y=0

                                                     ⇒    6x-y+8=0

    The Standard Form is as follows:                                                                        

                                                      6x-y=-8   

How to Find the y-Intercept of line Equation:

We can find the y-intercept when x=0, it is a point where the line crosses the y-axis. Now insert the values in the equation of the line calculator, we find the values:

                                          y = 3x – 7

  Using the equation y = 3x – 6, keep x=0, to find the y-intercept:                                   

                                           y = 3(0) – 7

                                           y= -7

The y-intercept is “-7”.

How to Find the X-Intercept of line Equation:

The x-intercept of a line is the value of x when y=0. It is the point where the line crosses the x axis of the cartesian coordinates. We can write an equation of the line that passes through the points y=0 as follows:

Using the equation: 

                                            y = 3x – 6, put y=0 

To find the x-intercept.

                                                  0 = 3x – 6

                                                   3x = 6

The x-intercept is 2 of the slope-intercept form of line equation is:

                                                   x = 2

The slope of the Parallel lines:

When we are finding the slope of one line then there will be the same values of the parallel line as they have the same values of “m”. So they never intersect with each other.

The slope of Perpendicular Lines:

Perpendicular lines will have a slope equal to the negative inverse of the known slope. 

We can find an equation of the line which is perpendicular to the other. These lines from a 90° angle when they intersect.

How to Find the Equation of a Line Perpendicular to Others?

Consider a line with a slope of -5.
Then what is the slope of the line perpendicular to it?

• First, take the negative of the slope of your line
  -(-5) = 5
• Second, take the inverse of that number.
• 5 is a whole number, its denominator is 1.
• The inverse of 5/1 is 1/5.
• The negative inverse of -5 is a slope of 1/5.
• The slope of the perpendicular line is 1/5.

The equation of a line that passes through two points and is perpendicular to each other has slope “5” and ⅕ respectively.

Working on the Equation of a Line Calculator:

You can find the equation of the line point-slope form, slope-intercept form and standard form additionally we are able to find the equation of a line with two points on the Cartesian coordinates.

Input:

• Insert the values by choosing the drop-down menu.
• Choose Two-point, One point, or a y-intercept from list 
• Insert values
• Press, the calculate button

Output:

The line equation from two points calculator unveils through some solution of all the forms of the equation of the line. The graphical representation depicts us to understand the whole concept easily.

• A detailed solution is displayed.
• A graphical representation is also represented

FAQs:

How do you graph the equation of a line?

You can graph an equation by finding the y-intercept = c of the equation y = mx + b and slope of line.

What is the Y-intercept of the straight line?

It is a point where the straight line cuts down the y-axis, and x=0, when y=mx+c. Then c is the y-intercept of the equation.

References:

From the source of content.byui.edu:Point-Slope Form of a Line, Additional Resources

From the source of Wikipedia:  Line, Definitions versus descriptions, In Cartesian coordinates

From the source of chilimath.com: Ways to Graph, X, Y coordinates