Area Calculator

Make use of this free shaded area calculator that lets you find the area of any random geometrical shape within a couple of clicks.

So it’s time to move on and see how to calculate area of figures either manually or by using this land measurement calculator.

Stay with it!

What Is The Area of The Figure?

A particular measurement of a two dimensional figure that shows its actual size is known as the area of the closed geometric figure. You can find surface area of three dimensional shapes by our surface area calculator.

Unit of Area:

In System International, the area is expressed in the units of metre squared \(\left(m^{2}\right)\). If you wish to determine the area with this plot area calculator land, you will always obtain the answer in standard unit measurements.

How To Find Area?

In the following section, we will be taking you through the glimpse at the formulas to calculate areas of anonymous shapes. So fasten your seatbelt as it is time to start the ride!

Square:

A square is a shape having equal measurements for its length and width. Get going to insert the values in the following equation if you are interested in determining the area of the square.

$$ \text{Square Area} = a * a = a^{2} $$

where;

a = Length of the square side

Rectangle:

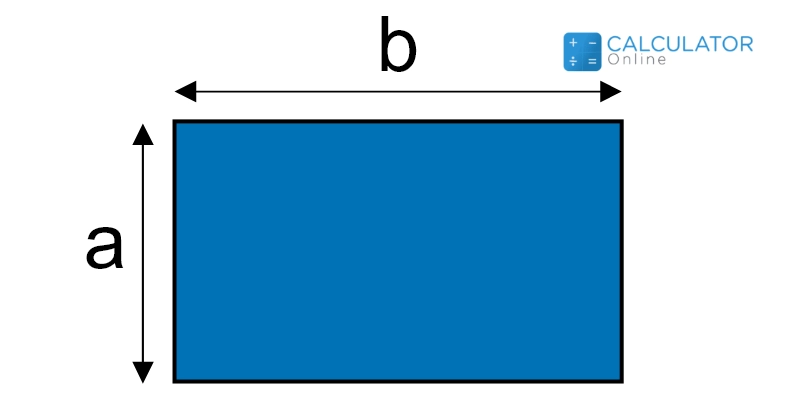
If you extend two opposite sides of a square up to equal lengths that are faced towards each other, then a figure obtained is termed a rectangle. The area measurement for a rectangle stone can be done either by using this area of a rectangle calculator or by employing the equation as under:

$$ \text{Rectangle Area} = a * b $$

Triangle:

Now to calculate area of a triangle, we have various postulates that must be encountered. And on the basis of different parameters used, the formulas also change as well and are given as per below:

When Height and Base are Provided:

$$ \text{Triangle Area} = \frac{b * h}{2} $$

If Two Sides And their Mutual Angle is Provided: SAS

$$ \text{Triangle Area} = 0.5 * a * b * sin\left(γ\right) $$

If Three Sides of Triangle are Given:

$$ \text{Triangle Area} = 0.25 *\sqrt{\left(a + b + c\right) * \left(-a + b + c\right) * \left(a – b + c\right) * \left(a + b – c\right)} $$

If Two Angles and Side Touching Them are Provided:

$$ \text{Triangle Area} = \frac{a^{2}*sin\left(β\right)*sin\left(γ\right)}{\left(2 * sin\left(β + γ\right)\right)} $$

The free triangle area calculator also make use of all these formula

Circle:

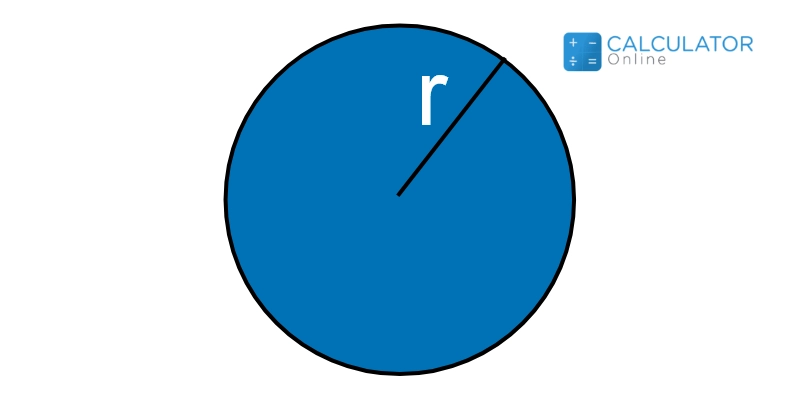
This is one of the most common and widely used figures in geometrical analysis. And without , it is nearly impossible to understand the laws of geometry. Coming to the point now, you can calculate area of a circle by using the formula:

$$ \text{Circle Area} = πr^{2} $$

The area calculation for any kind of circle can instantly be carried out with this find area calculator. Apart from this, you can make use of our another unit circle calculator if you are dealing with such a circle type and want to examine its all related parameters.

Semicircle:

It’s a daughter type of circle but being a little bi different in shape, the formula to find the area is as below:

$$ \text{Area of a Semicircle} = \pir^{2} $$

Sector:

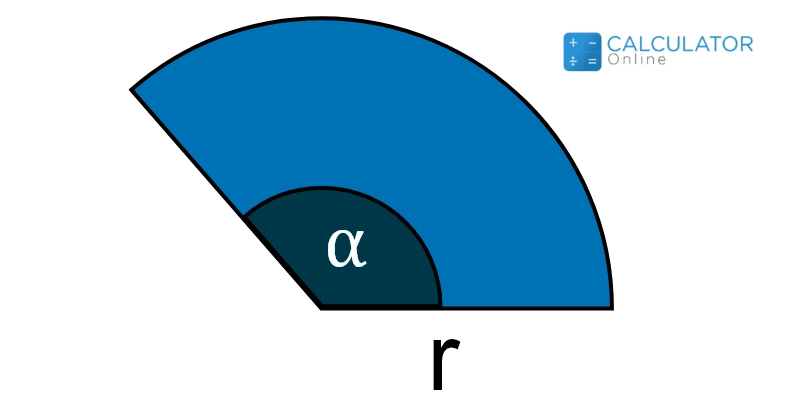
A sector is a measurement of a specific part of a circle. And if you wish to calculate its area, then you can follow up the formula given as:

$$ \text{Area of a Sector} = \frac{r^{2}*𝜶}{2} $$

You can also employ the area of a sector calculator to determine the area and other crucial parameters of a circle sector.

Ellipse:

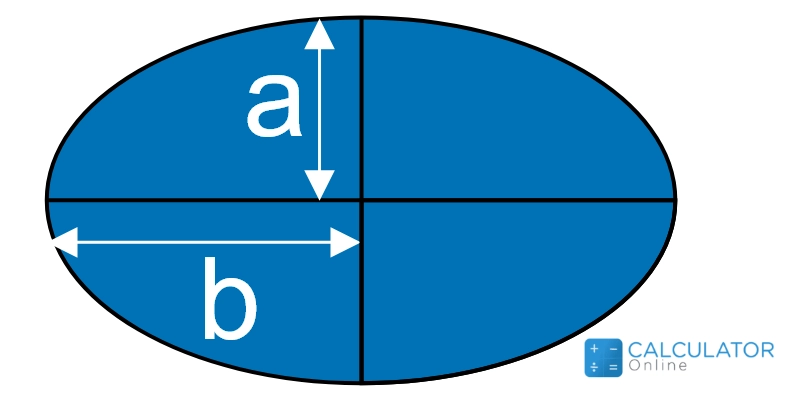
Now as you better know that both circle and ellipse are identical in shape. But when you encounter area calculation for ellipse, then you must consider the length of the major and minor axis instead of radius. This is given as:

$$ \text{Area of an Ellipse} = \pi*a*b $$

Trapezoid:

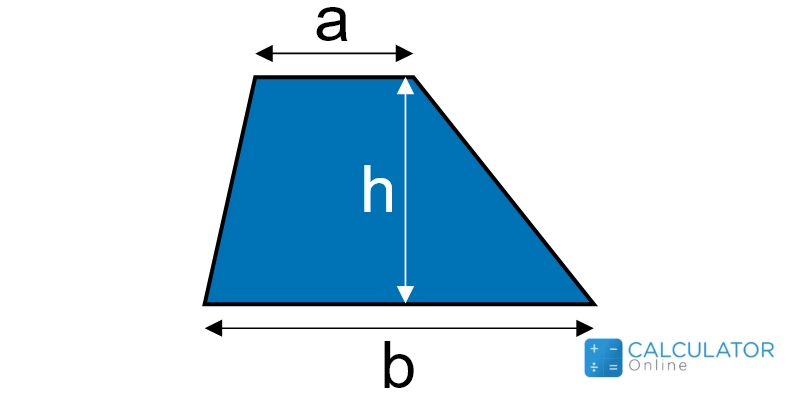
To find the area of a trapezoid, you need to recall the equation as:

$$ \text{Area of a Trapezoid} = \left(a*b\right)*\frac{h}{2} $$

Parallelogram:

Now here arise three different cases like that of triangle and are given as follows:

If height and Bass are Provided:

$$ \text{Area of a Parallelogram} = a*h $$

If Two Sides and Angle Between them are Provided:

$$ \text{Area of a Parallelogram} = a*b*sin\left(𝜶\right) $$

If Diagonals and Mutual Angle Is Given:

$$ \text{Area of a Parallelogram} = a*b*sin\left(θ\right) $$

Moreover, you can also explore a parallelogram by using the online parallelogram calculator.

Rhombus:

Get going through the area formulas to find the area of the rhombus as follows:

If Side and Height are Given:

$$ \text{Area of Rhombus} = a*b $$

If Diagonals are Given:

$$ \text{Area of a Rhombus} = \frac{\left(a*b\right)}{2} $$

If One Side and Any Angle Is Given:

$$ \text{Area of a Rhombus} = a^{2} * sin\left(𝜶\right) $$

Kite:

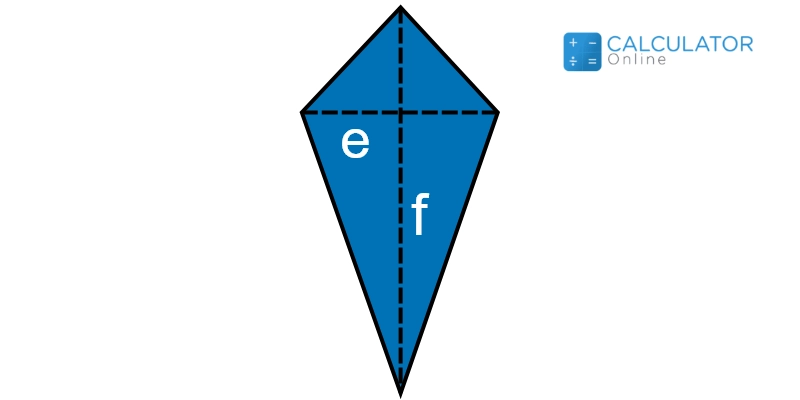
Here we have a couple of formulas that are used in certain condition where you are given with different parameters for area calculations:

If Diagonals are Given:

$$ \text{Area of a Kite} = \frac{\left(a*b\right)}{2} $$

If Two Sides and their Mutual Angle Is Given:

$$ \text{Area of a Kite} = a*b*sin\left(𝜶\right) $$

Regular Pentagon:

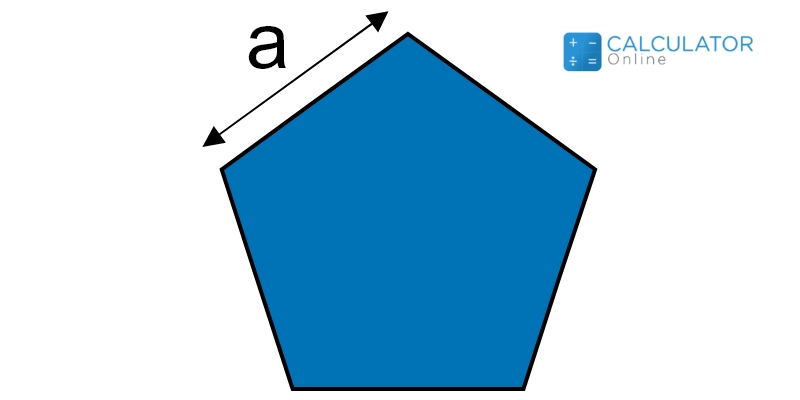
The following expression lets you calculate area of any pentagon:

$$ \text{Area of a Pentagon} = a^{2}*\frac{\sqrt{\left(25+10\sqrt{5}\right)}}{4} $$

where;
a represents the side length

Regular Hexagon:

Go by considering the equation mentioned as under to calculate area of a regular hexagon:

$$ \text{Area of a Hexagon} = \frac{3}{2} * \sqrt{3}*a^{2} $$

where;

a is the length of a hexagon side.

However, we recommend you utilise our free area of shaded region calculator to determine the area of a hexagon.

Annulus (Ring):

As you know annulus is a ring shaped figure. And in this kind of figure, we have a couple of circles, one having radius R and other having radius as r. Now you can calculate area of a shape like an annulus by subtracting the area of the smaller circle from that of the bigger one.

$$ \text{Area of an Annulus} = \piR^{2} – \pir^{2} $$

$$ \text{Area of an Annulus} = \pi\left(R^{2} – r^{2}\right) $$

Irregular Quadrilateral:

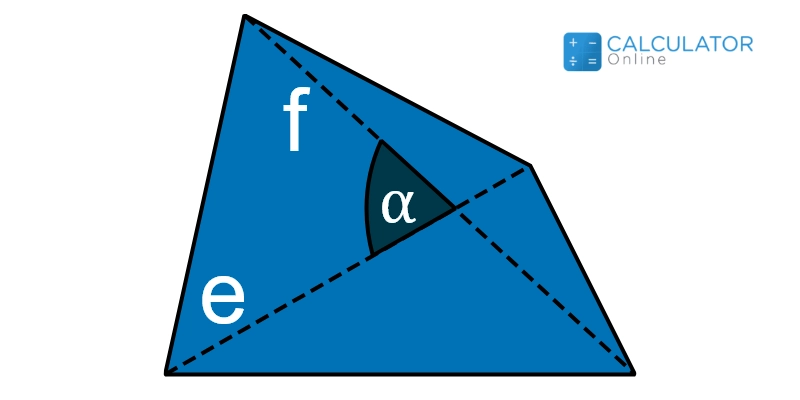
Like triangle area, the area calculation for quadrilateral can also be performed by using the various formulas. Among these, the most effective and handy is given as follows:

$$ \text{Area of a Quadrilateral} = a*b*sin\left(𝜶\right) $$

where;

a and b represent any two sides of the quadrilateral and alpha is the angle made in between them.

Regular Polygon:

Get going to explore the area of a polygon by considering the equation:

$$ text{Area of a Regular Polygon} = n*a^{2}*\frac{cot\left(\frac{\pi}{n}\right)}{4} $$

Also, we have developed the polygon calculator as well that lets you examine and calculate all particular parameters of a polygon accurately and flawlessly.

How Online Area Calculator Works?

Follow the guideline arranged below to use this land area calculator.

Input:

• From the top drop-down list, select the geometrical figure the area of which you are interested in calculating
• After you are done with your selection, write down the required parameters’ values in their designated fields
• Also, select the units for each entity entered
• Now hit the calculate button

Output:

The free area of a composite figure calculator does the following computations:

• Calculate area of common figures used in area calculations

FAQ’s:

How do you find the area of irregular figures?

Well, it’s pretty simple. What you need to do is to divide the irregular figure into common shapes as described in the content above. After you are done with this, you can calculate the areas of these geometrical shapes easily by this irregular shape area calculator rectangle. And once it is completed, simply add all tiny areas and you will get the overall area of the shape.

What quadrilateral has the largest area?

Among quadrilaterals, square is the one having the largest area calculations. You can also verify it by this free area of a rectangle calculator as rectangle is quite similar to square.

What is a 4 sided shape with no equal sides called?

A geometrical figure with no equal side is known as scalene quadrilateral. And you can find the area of this particular shape by employing our best find the area of the shaded region calculator.

How do I calculate the area under a curve?

Well, it’s very simple! What you need to do includes a couple of factors. One is the manual calculations of the area by indefinite integral simplification. The other one is the use of the area under the curve calculator that is the best way considered so far.

What is a 100 sided shape called?

It’s hectogon having 100 sides of almost equal lengths.

What shape has the largest area given perimeter?

Among all geometrical figures, the circle is the one having the largest area measurement with perimeter being provided.

What is the area of a cube?

Below we have the formula that helps you calculate the area of the cube:

Area of a cube = Length of side * 6

Conclusion:

No doubt it is hard sometimes to calculate the area of any irregular shape. This is because not everyone is capable of estimating the right dimensions of complicated figures or land areas. But to cope with the situation, we have developed this find the area calculator online so that you people may not feel any hurdle while calculating the area of the irregular figures.

References:

 From the source of Wikipedia: Geometry, Axioms, Objects, Congruence and similarity, Contemporary geometry, Applications, 

From the source of Khan Academy: Unit squares, Measuring area with partial unit squares, Creating rectangles

From the source of Lumen Learning: Area of Irregular Figures, Surface Area of Rectangular Solids, A Bit of Geometry, Similar Triangles

Other Languages: Výpočet Plochy, Flächenberechnung, Calcolo Area, 面積計算, 면적 계산, Kalkulator Powierzchni, Calculo de Área, Calcular Area, Alan Hesaplama.