Voltage Drop Calculator

Calculate the overall drop in voltage for DC and AC circuits with the voltage drop calculator. The tool uses the NEC and Wire Resistance equations for power loss across a load connected in a single-phase and three-phase supply system and tells you the actual potential fall in the whole circuit. In addition to that, you may also get the wire cross-sectional area, resistance, and voltage across terminals with the calculator.

What Is Voltage Drop?

“It is the overall loss of the voltage due to the internal impedance of the circuit”

Pictorial Representation:

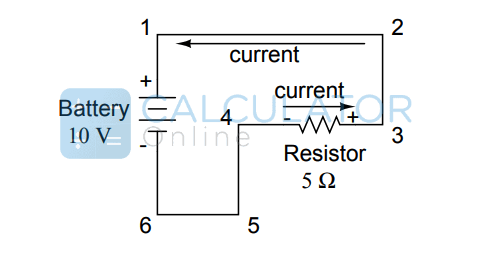
In the above figure, we have a potential voltage applied to a circuit network containing only a resistance. Now when the current passes through the resistance, there will be ampacity and drop in voltage from one end to the other end of the resistor. This drop can be instantly determined by using the voltage calculator above.

Types of Voltage Drop:

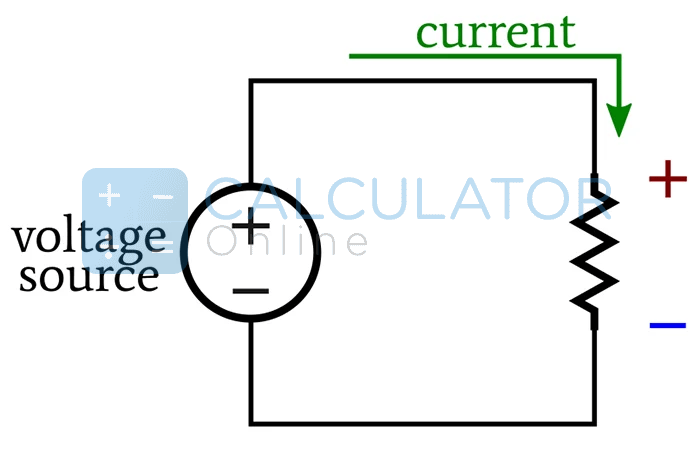
According to the IEEE (Institute of Electrical and Electronics Engineers), there are two types of voltage drop.

Positive Voltage Drop:

A positive drop occurs when the electronic current flows through the circuit.

Negative Voltage Drop:

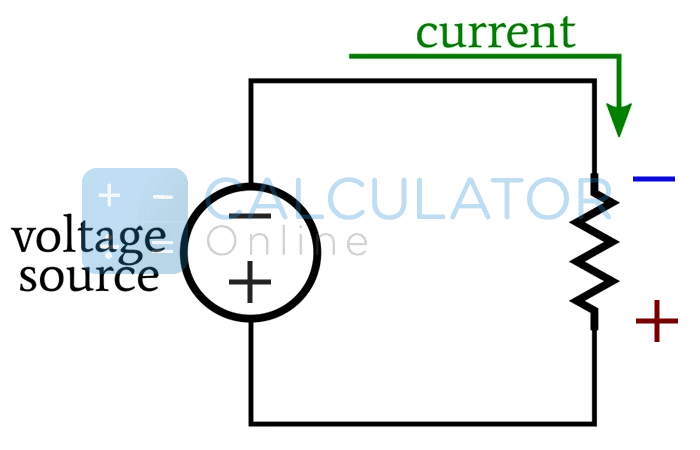
This voltage drop usually occurs in a circuit when there is a conventional current flowing through it.

Voltage Drop Equations:

Voltage Drop Formula for Single Phase:

\(V_{drop\left(V\right)} = I_{cable\left(A\right)} * R_{wire\left(ohms\right)}\) \(V_{drop\left(V\right)} = I_{wire\left(A\right)} * \left(2 * L_{\left(ft\right)} * \frac{R_{wire\left(\frac{Ω}{kft}\right)}}{1000_{\left(\frac{ft}{kft}\right)}}\right)\) \(V_{drop\left(V\right)} = I_{wire\left(A\right)} * R_{wire\left(Ω\right)}\) \(V_{drop\left(V\right)} = I_{wire\left(A\right)} * \left(2 * L_{\left(m\right)} * \frac{R_{cable\left(\frac{ohms}{km}\right)}}{1000_{\left(\frac{m}{km}\right)}}\right)\)

Voltage Drop Formula for Three Phase:

\(V_{drop\left(V\right)} = \sqrt{3} * I_{wire\left(A\right)} * R_{wire\left(Ω\right)}\) \(V_{drop\left(V\right)} = 1.732 * I_{wire\left(A\right)} * \left(L_{\left(ft\right)} * \frac{R_{wire\left(\frac{Ω}{kft}\right)}}{1000_{\left(\frac{ft}{kft}\right)}}\right)\) \(V_{drop\left(V\right)} = \sqrt{3} * I_{wire\left(A\right)} * R_{wire\left(Ω\right)}\) \(V_{drop\left(V\right)} = 1.732 * I_{wire\left(A\right)} * \left(L_{\left(m\right)} * \frac{R_{wire\left(\frac{ohms}{km}\right)}}{1000_{\left(\frac{m}{km}\right)}}\right)\)

Whatever the phase is, the calculator will take a couple of seconds to display the actual loss in the voltage transmission.

Diameter of the Wire:

For a cable having a diameter in inches and n gauges:

\(d_{n\left(in\right)} = 0.005 inches * 92^{\frac{\left(36-n\right)}{39}}\)

And When The Diameter Is In Millimeters:

\(d_{n\left(mm\right)} = 0.127 mm * 92^{\frac{\left(36-n\right)}{39}}\)

Cross Section Area of Wire:

\(A_{n\left(kcmil\right)} = 1000 * d_{n}^{2} = 0.025 in^{2} * 92^{\frac{\left(36-n\right)}{19.5}}\) \(A_{n\left(in^{2}\right)} = \left(\frac{\pi}{4}\right) * d_{n}^{2} = 0.000019635 in^{2} * 92^{\frac{\left(36-n\right)}{19.5}}\) \(A_{n\left(mm^{2}\right)} = \left(\frac{\pi}{4}\right) * d_{n}^{2} = 0.000019635 mm^{2} * 92^{\frac{\left(36-n\right)}{19.5}}\)

Resistance By The Wire:

\(R_{n\left(\frac{Ω}{kft}\right)} = 0.3048 * 10^{9} * \frac{ρ\left(Ω.m\right)}{25.4^{2} * A_{n\left(in^{2}\right)}}\)

FAQ’s:

What Causes a Voltage Drop?

When the potential at the end of the wire gets higher than the potential at the start, then it gives rise to the potential voltage drop.

Does Voltage Drop Over Distance?

The distance is directly proportional to the resistance and when the resistance increases, the Voltage drop will also get maximum.

How Do You Prevent Voltage Drop?

• The temperature of the conductor must be reduced to enough
• The length of the conductor should be decreased
• Reduce the power load voltage connected to the circuit
• Increasing the number of the conductors connected in the circuit

You must keep checking the drop with the calculator to analyze better.

How Much Voltage Drop Is Acceptable?

The maximum voltage loss calculation that could be tolerable between a feeder conductor to the farthest load connected in series or parallel must not exceed the value of 5%.

What Is The Maximum Voltage Drop In a Domestic Property?

• Single phase = 230.4 V
• Three phase = 398.4 V

American Wire Gauge (AWG) Sizes: